Doses

DEFINITIONS OF SOME QUANTITIES USED IN MEDICAL X-RAY CLINICS

X-ray tube output, W_d,U:

W_{\scriptscriptstyle d,U}= \frac {K_{\scriptscriptstyle d,U}}{I_{\scriptscriptstyle R} \cdot t}

where: K_d,U – is the air kerma measured on the central beam axis at distance d from x-ray tube focus for voltage U, I_R∙t – is tube-current exposure-time product. In the SI system x-ray tube output is expressed in units of [Gy/C] or [Gy/A·s], usually the tube output is expressed in units of [mGy/mA·s].

Kerma-area product, KAP:

\textit{KAP}=\int_A K_a ~ dA

simplified formula: KAP=K_a*A

where: K_a – is the air kerma measured on area A. In the SI system, the air kerma-area product is usually expressed in units of [Gy·m²]. For x-ray radiation KAP is equivalent of DAP (dose-area product).

Kerma-length product DLP:

\textit{DLP} = \int_L D \left ( z \right )~dz

where: D(z) – is the absorbed dose distribution alongside the axis of rotation of a CT scanner (z-axis) for a single rotation of a CT scanner (360˚). In the SI system, DLP is expressed in units of [Gy·m].

Computed tomography dose index, CTDI₁₀₀:

\textit{CTDI}_{\scriptscriptstyle {100}} = \frac 1 {N \cdot s} \int^{+50 mm}_{-50 mm} D \left ( z \right )~dz

where: D(z) – is the absorbed dose distribution alongside the axis of rotation of a CT scanner  (z-axis) for a single rotation of a CT scanner (360˚), s – nominal slice thickness, N – number of slices per single rotation. In the SI system CTDI₁₀₀ is expressed in units of [Gy].

Weighted CT dose index, CTDI_w:

\textcolor{black}{\textit{CTDI}_w = \frac 1 3 \textit{CTDI}_{100,A} + \frac 2 3 \textit{CTDI}_{100,P}} (fantomy PMMA);

where: A – is the axis of rotation of a scanner, P – 10 mm slice thickness from the surface of the phantom. In the SI system, CTDI_w is expressed in units of [Gy].

CT pitch factor, CTPF:

\textit{CTPF} = \frac {\varDelta d} {N \cdot s}

where: Δd – is the distance moved by the patient coach per a single rotation of the scanner, N, s – see above. CTPF is a dimensionless quantity.

Volume averaged weighted CT dose index, CTDI_vol:

\textit{CTDI}_{vol} = \frac {\textit{CTDI}_w} {\textit{CTPF}}

In the SI system, CTDI_vol is expressed in units of [Gy].

\textit{DLP} = \textit{CTDI}_{vol} \cdot L

where: L – total scan length during the examination

Entrance surface dose, D_ent:

D_{\scriptscriptstyle ent} = K_{\scriptscriptstyle E} \cdot B

where: K_E – is air kerma measured at the surface of patient’s skin where the beam enters the patient, B – backscatter radiation from the patient. In the SI system, D_ent is expressed in units of [Gy].

Table 1: Rough estimates of backscatter factor for the voltage around 70 kV depending on filtration an beam field size (on the basis of IAEA training materials)

Entrance skin dose, ESD:

\textit{ESD} = K_{\scriptscriptstyle E} \cdot B \left ( \frac \mu \rho \right )^{skin}_{air}

where K_E – is air kerma measured at the beam axis on the patient’s skin, B – backscatter factor from the patient, (μ/ρ) – mass absorption coefficient skin-to-air. In the SI system, ESD is measured in units of [Gy].

Table 2: Mass absorption coefficient for skin, air and skin-to-air for selected voltages (on the basis of ICRU-44 and J.H Hubbell and S. M. Seltzer)

RELATIONSHIPS BETWEEN SOME QUANTITIES USED IN X-RAY CLINICS

Relationship between kerma (dose) and distance:

K_{\scriptscriptstyle 1} = K_{\scriptscriptstyle 2} \left ( \frac {r_{\scriptscriptstyle 2}} {r_{\scriptscriptstyle 1}} \right )^2

where: r_i – is the distance from the tube focus, K_i – is the air kerma (dose) at distance r_i

Relationship between kerma and kerma-area product (KAP):

K_{\scriptscriptstyle S} = \frac {\textit{KAP}} {A_{\scriptscriptstyle S}}

where: KAP – is the measured kerma-area product, A_S – radiation field in the plane perpendicular to the beam axis at point S, K_S – kerma at point S. For x-ray radiation KAP is equivalent to DAP.

Relationship between beam field sizes:

A_{\scriptscriptstyle 1} = A_{\scriptscriptstyle 2} \left ( \frac {r_{\scriptscriptstyle 1}} {r_{\scriptscriptstyle 2}} \right )^2

where: r_i – is the distance from the tube focus, A_i – beam field size at distance r_i

Calculating kerma at point E based on measured tube output:

K_{\scriptscriptstyle E}  = W_{\scriptscriptstyle d,U} \cdot I_{\scriptscriptstyle R} \cdot t \cdot \left ( \frac {r_{\scriptscriptstyle d}} {r_{\scriptscriptstyle E}} \right )^2

where: W_d,U – is the tube output calculated in point d for voltage U, I_R·t – is the tube-current exposure-time product, r_d –  distance between the point where output was measured, W_d,U, and the tube focus, r_E – distance of the point where kerma K_E  was determined from the tube focus.

GENERAL ALGORITHM FOR CALCULATING ENTRANCE SURFACE DOSE AND ENTRANCE SKIN DOSE

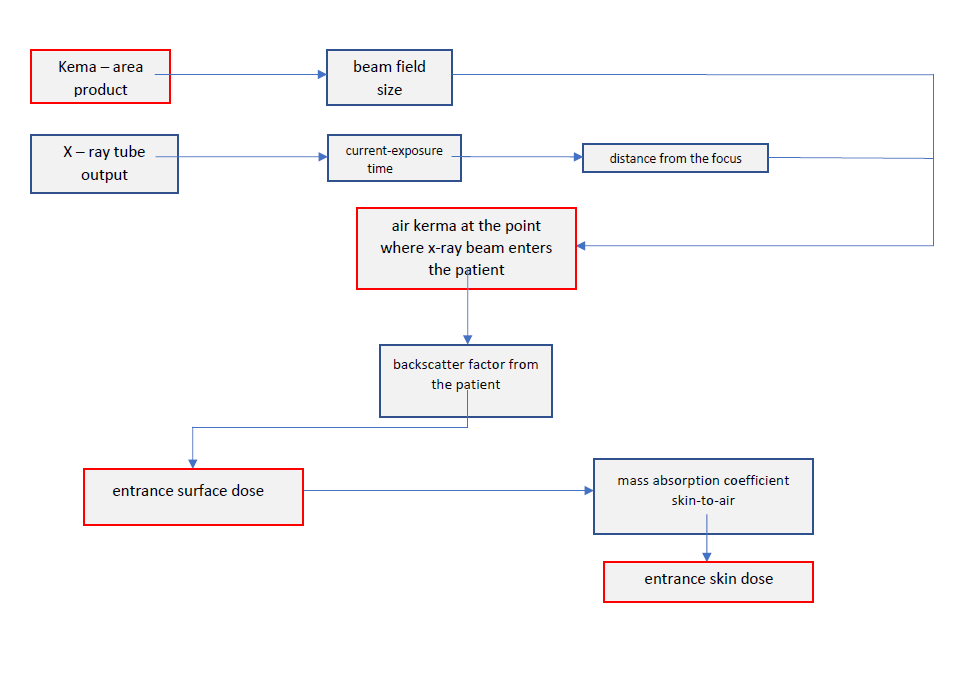
Simplified calculations:

• https://www.fetaldose.org/
• https://www.jednostek-miary.info/
• http://www.radprocalculator.com/

For more information see:

1. H. Aichinger, J. Dierker, S Joite-Barfuss, M.Saebel: Radiation Exposure and Image Quality in X-ray Diagnostic Radiology (Springer 2004, ISBN 3-540-44287-1) link
2. Entrance skin dose estimates derived from dose-area product measurements in interventional radiological procedures, B. J. McParland, BJR, 71 (1998), 1288 – 1295 link
3. A study of patient radiation doses in interventional radiological procedures B.J. McParland, BJR, 74 (2001), 727 – 734 link
4. Differences in effective dose estimation from dose-area product and entrance surface dose measurements in intravenous urography E. Yakoumakis at al, BJR,, 74 (2001), 920 – 925 link